Museum of Electric Lamp Technology

发光基础概念

• 光是电磁波, 是信息的载体。频率、波长、相位、振幅、偏振、 模式等等 (信息技术基础Ce3+掺杂荧光粉，存在两种解)
• 光由光子(粒子)构成, 是能量的载体 (能源技术基础)
• 光与物质发生电磁相互作用, 反映物质内部的信息, 并改变物质的属性 (物质科学技术基础) ——李志远(华南理工)
• From a puzzling experimental result to its interpretation, to the development of a general concept, and finally, to the prediction of new phenomena or the development of a new method - this is the line which can be traced in many of his studies. (P.P. Feofilov and the Spectroscopy of Activated Crystals)

吸收和发射

我们可以这样理解【吸收截面 】，一个粒子A飞向粒子B，这个时候B展开了"一把伞"，这个伞就可以看作是吸收(反应)截面，只要粒子A打在这个伞上，两个粒子就可以发生反应。显然，伞的面积越大，截面就越大，反应就越容易发生。另外从这里也可以看出，吸收截面的量纲是面积。[袁岚峰] 

吸收截面比较学术的说法：吸收截面可以理解为从核反应截面衍生而来。首先我们来理解【核反应截面】。当具有一定能量的粒子轰击拔和靶核时，可能发生各种类型的核反应，每种核反应都有一定的几率，为了描述这种反应几率的大小，引入了核反应截面的概念。其物理意义是表示一个粒子入射到单位面积内只含一个靶核的靶子上发生反应的几率。核反应截面用下面的式子表示:$$\sigma=N^{\prime} /\left(I N_{\mathrm{s}}\right)$$也就是单位时间内发生的核反应数除以单位时间内入射的粒子数以及单位面积内的靶核数。术语”截面”被用于物理学量化某一特定的粒子与粒子相互作用的概率，例如散射，电磁波吸收等。

注：
(1) 进一步延伸，如果过来的光束方向和现在的粒子的吸收截面垂直，假设光束里面含有一个光子，那么该粒子的吸收截面除以光束的截面即为吸收这个光子的几率。
(2) 以激光介质为例子，可描述为将激光介质中每个吸收光强的粒子视为一个小光删，它将入射到介质中的光挡掉, 而吸收截面就是这个小光删的横截面积。
(3) 微分散射截面：\(\displaystyle\frac{d \sigma}{d \Omega}\)是对散射截面关于空间立体角求导的结果，由于空间立体角无量纲，所以导数依然具有面积的量纲。总的散射截面\(\sigma\)等于微分散射截面\(\displaystyle\frac{d \sigma}{d \Omega}\)对全空间立体角的积分\(\sigma=\displaystyle\int \frac{d \sigma}{d \Omega} d \Omega\)。参考如何理解微分散射截面?-知乎

对于电磁波的吸收，存在这样的关系：$$\frac{d N}{d x}=-N n \sigma_{a b s}$$其中\(n\)是单位体积的吸光分子的数目(比如单位体积二价Sm离子的浓度)，它和吸收截面的乘积\( n \sigma_{a b}\)就是吸收系数\(\alpha\)。根据\(  I=I_{0} \exp (-\alpha L)\)这个公式，【吸收系数】的含义就是光通过\( 1 / \alpha\)(单位是cm)长度后，光的强度变为初始强度的\(1 / e\)。从另一个方面来说，如果入射光强度一定，而且作用的物质不变(光通过的路径，以及吸光分子的浓度不变)，那么透射光和入射光强度比值的\(\ln\)值和吸收截面成正比。吸收截面和吸收系数，都和波长有关。

注：
(1) 无论是吸收系数还是吸收截面，都和波长有关，所以上式其实可以写成$$I(\nu)=I_{0}(\nu) \exp (-\alpha(\nu) L)$$(2) [Optically stimulated detrapping during charging of persistent phosphors-OME-2016]文章中的\(p\) 既不是吸收截面，也不是吸收系数，而是“吸收截面×光通量”；
(3) 温度升高会造成吸收峰的展宽，随着温度的升高，有的激发波长的吸收可能变大了，有的反而变小了。
(4) 温度升高会造成吸收峰的展宽，可能使得reabsorption增强，QE降低。[Andries_CM_2009]

【发射截面】以激光介质为例子，将激光介质中每个发光粒子视为小光源，所发光强度即为该粒子所在处的光强，而发射截面就是此光源的横截面积。发射截面可以评估材料的(激光器)阈值和斜率效率。

变温吸收(反射)
Temperature dependent reflection spectroscopy is useful to study: [Thomas Jüstel-PPT]

•  body color shift
•  band gap shift
• QE drop with temperature 

 

朗伯比尔定律

【吸光度】(absorbance，也有的叫【optical density】)$$A=-\log _{10} \frac{I_{t}}{I_{0}}=\log _{10} \frac{1}{T}=K \cdot l \cdot c$$这个公式的物理意义是一束平行单色光通过非散射的均匀介质时，吸光度和光径长度以及溶液浓度成正比。注意如果这里的\( c\)的选择不同，可以是质量浓度，也可以是摩尔浓度，对应的消光系数\(I\)分别是质量消光系数\(\mathrm{L} \cdot \mathrm{g}^{-1} \cdot \mathrm{cm}^{-1}\)和摩尔消光系数\(\mathrm{L} \cdot \mathrm{mol}^{-1} \cdot \mathrm{cm}^{-1}\)。

适用条件：
(1) 入射光是平行、单色、垂直入射
(2) 溶液均匀，而且是非散射体系(肉眼看是澄清的)
(3) 溶液中的分子彼此之间没有相互作用(这要求为稀溶液，低于0.01摩尔每升)
(4) 入射光不引起溶液中反应的发生。
(5) 高浓度时，吸光粒子之间的平均距离减小，粒子间的电荷分布相互影响作用，那么吸收系数也会发生变化，导致偏离比尔定律。

上述公式其实和\(I=I_{0} \exp (-\alpha L)\)是等价的(写成\(I=I_{0} \exp (-\sigma L n)\)更直观地于上面的式子对应)。对于同一个溶液来说，消光系数正比于这里的吸收截面，也就是\(K \sim \sigma\)。二者的单位不一样，吸收系数的单位是\(  \mathrm{cm}^{-1}\)，，但是背后的物理含义是一致的，都是用来表征同一个性质的大小。

\(\log _{10} \displaystyle\frac{I_{t}}{I_{0}}=-K \cdot l \cdot c\)和\( \ln\displaystyle\frac{I}{I_{0}}=-\sigma Ln\)，其实就是左右两侧同时乘以一个常系数。参考换底公式$$\ln x=\log _{e} x=\frac{\log _{10} x}{\log _{10} e}$$

振子强度

振子强度的定义：实验跃迁的几率 / 理想情况下谐振子的跃迁几率
允许跃迁，振子强度很大，比如Ce³⁺的吸收

 

寿命

Mono-exponental decay
(其中\(N^*(t)\)表示激发态粒子的数目)  [Jonas-course-PhD] $$\begin{array}{l} { dN^*(t) }/{ dt }=-\omega^{\text{rad}}_{1\rightarrow 2}(N^*(t)) \\ N^*(t=0)=N_0 \end{array}$$解为\(N^*(t) =N_0\text{exp}({-\omega^{\text{rad}}_{1\rightarrow 2}\cdot t})=N_0\text{exp}({ -t }/{ \tau } )\)所以发光强度随时间的变化关系为$$I(t)= -\displaystyle\frac{ dN^*(t) }{ dt }=\omega_{1 \rightarrow 2}^{\text{rad}}N_0\exp \left(\frac{-t}{\tau}\right)=I_0\exp \left(\frac{-t}{\tau}\right)$$(1) The value of \(\tau\) depends strongly on the tyle of electronic transition
(2) shows dependence on emission energy(color), like CaS:Eu²⁺(red, 715 ns) and SrGa₂S₄:Eu²⁺(green, 450 ns)
(3) and depens on the medium's refractive index (local field effect), like SrSi₂O₂N₂:Eu²⁺ and SrGa₂S₄:Eu²⁺
(4) 这里的\(\omega_{1 \rightarrow 2}^{\mathrm{rad}}\)包含了通过辐射跃迁回到基态，以及非辐射跃迁回到基态的情况，两种情况可以简单的叠加处理，如果处于激发态的电子没有回到基态(比如被trap捕获)，那么就不是简单的叠加为一个整体的\(\omega \)，大概率是双指数的情况。
(5) 注：我们在推导基于Single barrier model的热稳定性的时候，将和温度有关的非辐射跃迁和温度无关的辐射跃迁几率是直接叠加为一个总的跃迁的，这是默认对应于同一类发光中心，否则的话，就是双指数了。

Bi-exponential decay 

$$I(t)=I_{1} \mathrm{e}^{-t / \tau_{1}}+I_{2} \mathrm{e}^{-t / \tau_{2}}$$其中\(\tau_{1}\)和\(\tau_{2}\)适合材料有关的本征的物理量，而\(I_{1}\)和\(I_{2}\)的大小受测试条件影响，只有\(I_{1}/I_{2}\)本身才有物理意义。

The fraction in the total emission intensity assigned to each \(\tau_{i}\) component: $$f_{i}=\frac{\displaystyle\int I_{i} \mathrm{e}^{-t / \tau_{i}} \mathrm{~d} t}{\displaystyle\int I(t) \mathrm{d} t}=\frac{I_{i} \tau_{i}}{I_{1} \tau_{1}+I_{2} \tau_{2}} \quad(i=1,2)$$双指数衰减是两种独立发光衰减过程的(线性)组合，假设\(I_1\)的发光来自state-1，\(I_2\)的发光来自state-2，对于这两个状态的退激发过程，均同时考虑辐射跃迁和非辐射跃迁。那么在脉冲激发后的瞬间，处在state-1的粒子数目为\(A_1\)，处在state-2的粒子数目为\(A_2\)。

对于state-1，\(  N_1^{*}(t)=A_1 \exp(-\omega_{\text{rad-1}} t--\omega_{\text{rad-2}}t)\)对应的发光强度$$I_1(t)=-\displaystyle\frac{d N^{*}(t)}{d t}\cdot \frac {{\omega_{\mathrm{rad}-1} } }{{\omega_{\mathrm{rad}-1} } +{\omega_{\mathrm{nrad}-1} } }=A_1{\omega_{\mathrm{rad}-1} }\cdot\text{exp}({-{\omega_{\mathrm{rad}-1}t } -{\omega_{\mathrm{nrad}-1} t}} )$$ 同理可以得到\(I_2(t)=A_{2} \omega_{\mathrm{rad}-2} \cdot \exp \left(-\omega_{\mathrm{rad}-2} t-\omega_{\mathrm{nrad}-2} t\right)\) 各自指前因子和对应寿命的乘积即$$A_{i}\omega_{\mathrm{rad}-i}\cdot \tau_{i} =\frac { A_{1}\omega_{\mathrm{rad}-i} }{\omega_{\mathrm{rad}-i}+\omega_{\mathrm{nrad}-i} }$$显然从公式中可以看出表示的是\(A_i\)个处于state-i激发态的粒子，有多少个通过辐射跃迁的方式回到了基态，因此通过加和就可以求总的通过发光回到基态的粒子的数目。
【指前因子】表示的脉冲激发后的瞬间存在该激发态上的粒子的数目，乘以其辐射跃迁的速率，注意最终的测试结果可能将数据比例放大，但是不同成分的指前因子的比例是不变的。

除了双指数，还有三指数等等，更一般地写作\(I(t)=\displaystyle\sum_{i} \alpha_{i} \exp \left(-t / \tau_{i}\right)\)，平均寿命表示为$$\tau_{\mathrm{avg}}=\Sigma \alpha_{i} \tau_{\mathrm{i}}^{2} / \Sigma \alpha_{i} \tau_{\mathrm{i}}$$

荧光粉双指数衰减的例子

• SrAl₂O₄:Eu²⁺,Dy³⁺在370 nm下的绿光峰寿命，在100 K时是单指数(和稀土本征发光有关)，然后随着温度的升高，变为双指数(增加了一个fast decay component)，新增加的部分作者认为和trapping process有关。注意虽然出现了双指数，但是初始的和稀土本正发光有关的成分的寿命还是保持不变。[Philipe_PRB_2014]
• Eu²⁺处在两种格位，对应的发光峰有重叠，如果检测重叠区域的发光衰减寿命，那么就会是双指数。
• Eu³⁺处在两种格位，那么可以通过site-selective spectroscopy来测出不同的单指数衰减的寿命(理想情况)。对于普通的光谱寿命测试，由于激发光的单色性不好(或者说同时覆盖两种Eu³⁺格位的激发谱)，因此可能得到双指数的衰减，分别对应不同的Eu³⁺的格位(不同的对称性)，参考文献[JSSC-2005]。
• CaGd_2(1-x)Eu_2x(WO₄)₄中，Interestingly, we can consistently fit the thermal behavior of the decay for different dopant concentrations with only two types of Eu ions (i.e. isolated ones and those showing energy transfer), both characterized by their own specific thermal behavior.
  低掺杂浓度，Eu²⁺表现为本征的发光，寿命随温度变化不大，表现为孤立的发光中心，单指数衰减(忽略475 K的情况)；全掺杂，Eu³⁺表现为都可以实现energy transfer，所以也是单指数衰减；对于中等浓度掺杂，有部分Eu²⁺表现为孤立发光中心的特点，有部分Eu²⁺表现为可以实现energy transfer的特点。参考[OE-2014]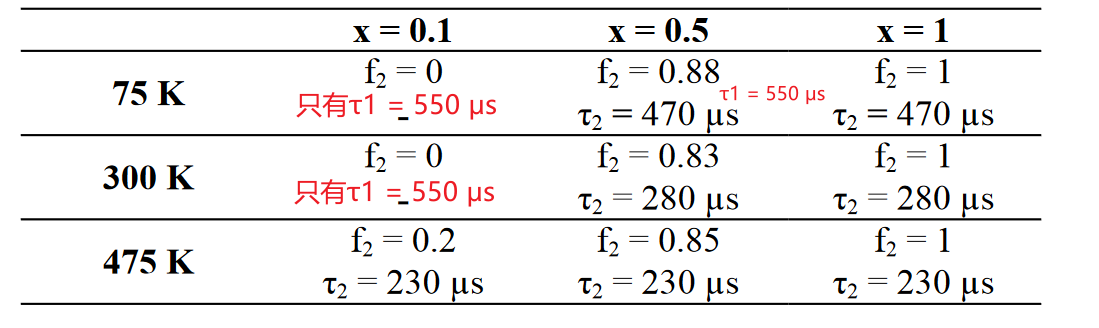
• Sr_1−xEu_xGa₂S₄, 也是低掺杂浓度下，表现为单指数衰减，随着掺杂浓度的升高，出现了双指数衰减的形式，其中一个指数保留了之前单指数衰减时的寿命，另一个指数的衰减寿命更短(对应的是non-radiative decay)，内量子效率也随着fast component decay的出现以及比例的增加而减小。At low concentration (x < 5%), the majority of the dopant ions can be considered as isolated centres, and the decay profile is described by a single exponential decay. For higher dopant concentrations, a second type of configuration is formed for which the lifetime is considerably shorter, presumably due to energy transfer between nearby Eu centres, and locally approaching the situation in EuGa₂S₄. 对于高掺杂浓度的样品，虽然同时有两种Eu存在，但是在低温下75 K二者表现出差不多的衰减特性，因此寿命测试上不可区分，即都可以用单指数拟合寿命曲线，而且和低浓度掺杂样品在75 K下的寿命差不多。参考[Jonas—OM-2012]。

Molecules数衰减的特点

• Typically, molecules exhibit nicely monoexponential decay. This is because molecules, especially dyes, are more well-behaved
• Molecules can deviate from monoexponential behavior for a few reasons:
  • Intramolecular charge transfer
  • Excimer formation (excited dimer)
  • Solvent effects

Nanomaterials数衰减的特点

Nanomaterials, on the other hand, rarely exhibit well-behaved monoexponential decay. 常见的原因如下

• Quantum dots (QDs) have 1000's of atoms (compared to handful for molecules), thus more states that can lead to compete de-excitation pathways.
• Surface states play a huge role in many QDs for adding emissive and nonemissive pathways. 比如量子点表面没有被钝化(Passivation)，那么处在表面的原子可能有dangling bonds猝灭发光。
• Interplay of bright and dark states is higher.

Tips

• 高掺杂浓度下，重吸收明显，重吸收会导致寿命增加。It is well-known that reabsorption of emission gives rise to a longer decay time.[Andries_CM_2009]
• 低掺杂浓度下的寿命更能反映本征的寿命。 [Andries_CM_2009]
• 寿命也可以描述为发光强度衰减到初始强度\( 1/e\)所需的时间。
• 已知在\( t\)和\(t+\Delta t\)时间内有\( \Delta n\)个电子回到基态，这些粒子的寿命就是\( t\)，因此平均的寿命为\(\displaystyle\sum_{t=0}^{\infty} t \Delta n / n_{0}\)，写成积分的形式为(参考[固体发光讲义-许少鸿-P14])$$\text{average lifetime}=\frac{1}{n_{0}} \int_{0}^{\infty} t \alpha n_{0} e^{-\alpha t} d t=\alpha \int_{0}^{\infty} t e^{-\alpha t} d t=\alpha\left[\frac{e^{-\alpha t}}{\alpha^{2}}(-\alpha t-1)\right]_{0}^{\infty}=\frac{1}{\alpha}=\tau$$
• 常见发光中心的寿命[Thomas Jüstel-PPT]

线性微分算子与寿命

正如我们线性代数-2笔记中的有关Linear Differential Operators的内容，这里对\(N^{*}(t)\)求一阶导，其实就是将线性的一阶微分算子\(D\)作用在\(N^{*}(t)\)上，得到了一个\(-\omega \)倍的\(N^{*}(t)\)。在常规矩阵的特征值特征向量求解中，最常见的情况是矩阵算子作用在一个特征列向量上，得到的是该列向量的缩放版。这两种情况的不同之处在于，算子作用的线性空间不同。对于常规矩阵来说，作用的空间就是列向量空间，对于该列向量的各个元素不做约束，定义简单清晰；而线性微分算子作用的空间是\(e^{\lambda t}\)的线性组合组成的空间，对\(\lambda\)不做约束。对后者而言，只有当\(\lambda\)为特征值的时候，\(e^{\lambda t}\)正好就是算子\(D\)的特征函数(也可以看作向量)，即\(D(e^{\lambda t}) = \lambda e^{\lambda t}\)。注意特征函数缩放后仍然是特征函数。在已知\(D(e^{\lambda t}) = -\omega  e^{\lambda t}\)成立的前提下，必有\(-\omega =\lambda\)，于是特征函数为\(ce^{-\omega t}\)，其中\(c \)为常数系数，可根据初始条件确定。即有\(N^{*}(t)=ce^{-\omega t}\)。

 

量子效率

定义：For phosphor powder, an incident photon is either reflected or absorbed: [Jonas-course-PhD] $$N_{\text{inc}}=N_{\text{refl}}+N_{\text{abs}}=N_{\text{refl}}+N_{\text{rad}}+N_{\text{n-rad}}$$Internal QE: $$\text{QE}_i=\frac { N_{\mathrm{rad}} }{ N_{\mathrm{abs}} }=\frac { N_{\mathrm{rad}} }{ N_{\mathrm{inc}}-N_{\mathrm{refl}} }$$ External QE:$$\mathrm{QE}_{e}=\frac{N_{\mathrm{rad}}}{N_{\mathrm{inc}}}$$In terms of lifetimes: $$\mathrm{QE}_{i}=\frac { \tau^{\text{rad}} }{ \tau^{\text{rad}}+\tau^{\text{n-rad}} }=\frac { 1 }{ 1+\frac { \tau^{\text{n-rad}} }{\tau^{\text{rad}} } }$$内量子效率额外说明：[David-Acs Photonics-2017] $$\eta_{\text {in }}=N_{\text {em }} / N_{\text {abs }}$$where \(N_{\text{em}}\) and \( N_{\text{abs}}\) represent the number of emitted and absorbed photons, respectively. Be careful with the definition of \(N_{\text{abs}}\)
(1) at the level of the phosphor (in most cases)
(2) at the level of the luminescent ion.

EQE = ​吸收*IQE, 对荧光粉来说，增加掺杂浓度可以提高吸收的比例，但是IQE会下降(浓度猝灭)，所以there exists a trade-off between the increasing absorption (and emission) and a decrease in the internal quantum efficiency due to concentration quenching. [Philipe_JES_2011]

注：
(1) 最好不要用寿命计算量子效率。
(2) 如果吸收比例很低，那么可能出现内量子效率很高，但是外量子效率很低的情况。
(3) 影响外量子效率的吸收率，不仅与荧光粉材料有关，也和荧光粉的颗粒大小，表面形貌有关，甚至实际中的使用环境也有关。
(4) Even when a material has a high quantum - or conversion - efficiency, the energy efficiency is limited by the Stokes shift.
(5) 量子效率也和激发光的强度有关，参考David-Acs Photonics-2017
(6) Quantum yield and brightness-JL-Peter A. Tanner
(7) QE和QY的差异，不同领域(photoluminescence, photochemistry, photovoltaics)的定义也有差异，参见researchgate的问答以及爱思唯尔的资料。
(8) 光致发光量子产率的测量概要(百度文库)

 

(热)猝灭/热稳定性

Single barrier model  (\(E_{\text{Mott}}\)) 或者 Mott-Seitz model
Expression with Boltzman factor: $$\omega_{1 \rightarrow 2}^{\mathrm{n-rad}}(T)=s\text{exp}(\frac { -E_{\text{Mott}} }{ k_\text{B}T } )$$ Yields (其中\(I_0\)表示的是温度为绝对零度即非辐射跃迁强度为零的情况下的发光强度)$$I(T)=\frac{\omega_{1 \rightarrow 2}^{\text {rad }} }{\omega_{1 \rightarrow 2}^{\text {rad }} +\omega_{1 \rightarrow 2}^{\text {n-rad }} }I_{0}=\frac { I_0 }{ 1+\frac { s }{ \omega_{1 \rightarrow 2}^{\text{rad}} } \text{exp}(\frac{-E_{\mathrm{Mott}}}{k_{\mathrm{B}} T})}$$ $$T_{0.5}=\frac {E_{\text{Mott}} }{k_{\text{B}}\ln(\frac { s}{\omega_{1 \rightarrow 2}^{\text{rad}} } ) }$$Influence on lifetime: two decay mechanism:$$\begin{array}{l} \displaystyle\frac{d N^{*}(t,T)}{d t}=-\omega_{1 \rightarrow 2}^{\mathrm{rad}}N^*(t,T)-\omega_{1 \rightarrow 2}^{\mathrm{n-rad}}(T)N^*(t,T) \\ N^*(t=0) =N_0 \end{array}$$ Yields:$$N^*(t)=N_0\text{exp}{(-\omega_{1 \rightarrow 2}^{\mathrm{rad}}t-\omega_{1 \rightarrow 2}^{\mathrm{n-rad}}(T)t)}=N_0\text{exp}(-\frac { t }{ \tau^\text{rad} }-\frac { t }{ \tau^{\text{n-rad}}(T) } )=N_0\text{exp}(\frac {-t }{ \tau(T) } )$$Monoexponential decay maintained, but with 【effective lifetime】. 这里的\(\omega_{1 \rightarrow 2}^{\mathrm{rad}}\)和\(\omega_{1 \rightarrow 2}^{\mathrm{n}-\mathrm{rad}}\)的比值也是处在激发态的电子选择辐射跃迁还是非辐射跃迁的概率(比例)。

温度依赖PL强度和温度依赖寿命的联系
Temperature dependent lifetime (图片来源)
(1) In first order, the decay time of the luminescence is following a similar thermal behavior than the intensity quenching [Philipe_JES_2011]描述的也是上图。
(2) SrAl₂O₄:Eu²⁺,Dy³⁺中，370 nm激发下的蓝光，也有类似现象。This correlation between thermal quenching and luminescence lifetime can be expected, due to the increased probability of nonradiative decay paths at elevated temperature, thus lowering the emission intensity and reducing the luminescence lifetime values.[Philipe_PRB_2014]

Mott的single barrier存在的问题是什么？[Jonas course and PhD]
答：wavefunction的重叠使得3-4个声子导致的热猝灭成为可能，但是fit this model to QM toy model with barrier height of 14个声子能量，也就是说energy barrier wrongly reproduced。另外this model neglects tunneling.  这里存疑？

Struck-Fonger Model

Struck and Fonger have shown that the temperature dependence of a non-radiative process is accurately described by considering ground and excited state vibrational wave function overlap. According to the Struck–Fonger model, the non-radiative process occurs through tunneling (crossover) from a vibrational level of the excited state to a high vibrational level of the ground state. The tunneling rate, i.e., the non-radiative decay rate, depends on the wave function overlap of the vibrational levels involved. The tunneling rate will be faster for a larger overlap between the wave functions and when the vibrational levels are in resonance. [Quenching of the red Mn⁴⁺-Light-2018]

拓展：[Thermal Quenching Analyses of Eu²⁺-Activated Sr-Containing Sialon Phosphors Using the Thermally Activated Cross-Over Model-ECS-2017]

Mott-Seitz model和公式Struck-Fonger Model差别(公式差不多)：The two commonly used models are the simple Mott model of the activation energy and the more-sophisticated model of Struck and Fonger using the socalled single-configurational-coordinate model, which describes in a simplified way the interaction between the electronic center and the vibrating crystalline environment. [Springer Handbook of Lasers and Optics edited by Frank Träger]-P676

低温下的热猝灭

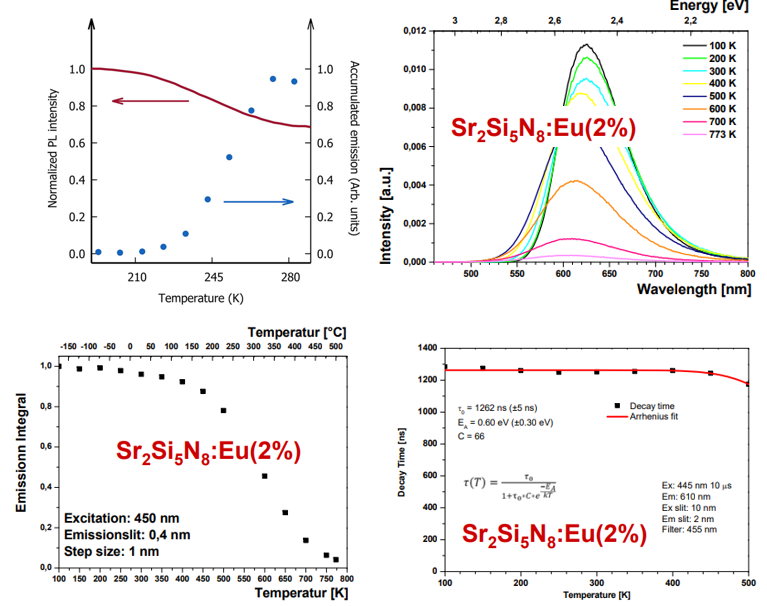
(1) thermal barrier for trap filling versus thermal barrier for PL
左上角的图来自[David-Acs Photonics-2017]，SrAl₂O₄:Eu²⁺,Dy³⁺为什么在220 K时，似乎也发生了热猝灭，发光强度降低，但是实际真实的热猝灭似乎又是从350 K开始的。220 K的发光强度的降低，其实因为trapping becomes thermally activated(从激发态的电子到导带需要能量)，所以激发态的粒子不是回到基态发光，而是将电子给了trap，另一方面，OSL的量子效率低，也会降低发光强度。所以这部分不是真实的热猝灭。
    在220 K用435 nm光激发样品，然后测TL，会发现没信号，说明存在thermal barrier for trap filling。注意thermal barrier for trap filling和thermal barrier for PL (导带模型)是不一样的，有联系(后者很大的，前者往往也表现明显)，但是不完全一样，因为临界温度(threshold)不同，前者是220 K，后者350 K，对应的能量显然也不同。[Philipe_PRB_2014]

(2) 另外三幅图来自[Thomas Jüstel-PPT]，他认为100-500 K时是Re-absorption导致了量子效率的下降，继续升高温度，IQE decreases due to IC and/or PI

存在energy transfer下的热稳定性$$ I_{\mathrm{PL}}(T)=\frac{I_0}{1+a \exp \left(-E_{\mathrm{q}} / k_{\mathrm{B}} T\right)}\left[1+A_{\mathrm{t}} \exp \left(-\frac{E_{\mathrm{t}}}{k_{\mathrm{B}} T}\right)\right] $$
参考文献：
(1) [JL-2004-Adachi] (2) [Nanoscale-2015-Chen xueyuan]

Thermal energy的三重影响(对Eu²⁺或者Ce³⁺掺杂荧光粉) [Philippe_JL_2012]

• PL thermal quenching
• photoconductivity
• trap filling
• 注：the thermal energy barrier is always smaller than the barrier determined from optical data in which relaxation effects that lower the barrier are not taken into account. [Fine structure in high resolution 4f⁷–4f⁶5d excitation and emission spectra of X-ray induced Eu²⁺ centers in LuPO₄: Eu sintered ceramics-JL-2019]

热稳定性 & 长余辉

• 热稳定性和长余辉此消彼长：我们常用的硅酸盐、铝酸盐长余辉荧光粉(Eu²⁺or Ce³⁺)，在室温下很容易实现可见光下(afterglow upon excitation into the lower 5d states. )的有效trap filling(非常低的温度可能也不行)，这也往往伴随着比较差的热稳定性。筛选Eu²⁺掺杂的长余辉材料(yellow-to-red emission that can be excited with blue-to-green light)，最好是PL热稳定性差的材料，这样更容易室温下用可见光填充traps，当然热稳定性也不能太差，否则trap filling存储的能量都以非辐射跃迁的形式释放了。[Philippe_JL_2012]
• 缺陷增强热稳定性-1：
  K₂BaCa(PO₄)₂:Eu²⁺通过计算认为绿色的那一段上升(zero thermal quenching)和氧空位有关。Drapping需要的能量大于thermal ionization所需的能量对于良好的热稳定性来说很重要。[夏志国_JACS_2018]
• 缺陷增强热稳定性-2：
  Na_3-2xSc₂(PO₄)₃: xEu²⁺荧光粉，高温下会出现的Na⁺ disorder。Te emission losses induced by nonradiative paths were effectively suppressed by a counter mechanism, which associated structural transformation leads to the formation of defect levels that can act as electron-trapping centres, favouring an energy transfer to the activator (Eu²⁺), which can counteract the quenching behaviour.[Jonas_NM_2017] 但是复旦大学闫世润老师给出了不同的解释，他认为该荧光粉分热稳定性不好。
  

热稳定性的机理解释
对于Ce³⁺掺杂荧光粉，存在两种解释[Tanabe_JPCC_2015]
(1) One is the thermally activated cross over from the 5d excited state to the 4f state. Thermally activated cross over is the nonradiative relaxation process from the 5d potential curve to the 4f ground potential curve through the crossing point in a configuration coordinate diagram.
(2) Thermal ionization is the thermally activated electron transfer process to the CB.
下图是三种热稳定性的解释：

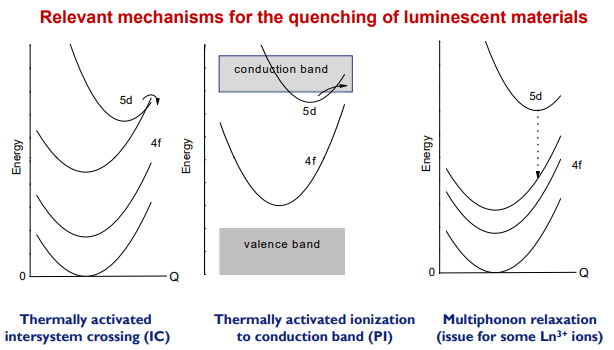
注：Tunneling to the ground state可能也是热猝灭的原因，参考[Thomas Jüstel-PPT]-P25/31，提到了YAG:Ce在低掺杂浓度下\(\mathrm{T}_{1 / 2}>300^{\circ} \mathrm{C}\)，这时猝灭原因是tunneling to the ground state。

热猝灭类型的判断：[Thomas Jüstel-PPT] 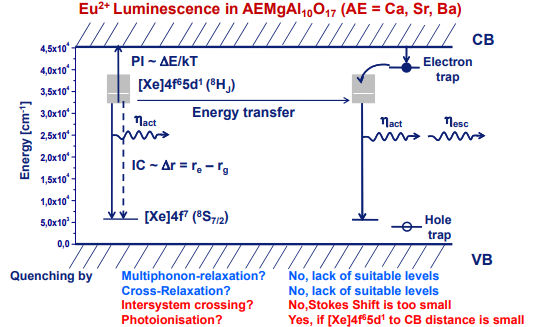f-f跃迁型稀土的热猝灭：由于是4f-4f 跃迁，跃迁几率小。这就使得离子在激发态停留时间延长，从而容易将激发能量传给另一个离子，并可能不断地传下去，直到被异种离子或晶格缺陷所吸收，造成猝灭。这样无辐射跃迁几率就会增大。同时不同稀土离子的发射能级下面，还有距离较近的能级，可以和上能级发生无辐射的能量交换，导致无辐射跃迁（对此下面还将有较多的讨论）。这也会降低发光效率。参考固体发光讲义-许少鸿

Tips:

• Quenching temperature: defined as the temperature where the emission intensity has dropped by 50 %.
• 对于高功率LED来说，荧光粉的热稳定性很重要，因为高功率意味着flux大，荧光粉工作温度高。YAG荧光粉就是热稳定性差，不太适合高功率LED。
• f-f emitter热猝灭机理：[Philipe_JES_2011]
  • phonon-activated crossing of the excited state and the charge transfer state
  • multi-phonon emission
• 荧光粉的热稳定性和掺杂浓度有关，比如YAG:Ce³⁺，低掺杂浓度下的热稳定性非常好(700 K)，但是实际商用的掺杂浓度其实比较高，而且猝灭温度很低(400 K)就有明显的发光强度的下降(这可以用thermally activated concentration quenching解释)。[Andries_CM_2009]
• 研究热猝灭原因，最好采用寿命作为研究对象(反映intrinsic quenching)，因为温度影响吸收的强度(the absorption strength is strongly temperature-dependent)，也会影响能量的migration和reabsorption，因此luminescence lifetime measurement are performed to provide better insight into the quenching temperature for Ce³⁺ emission。[Andries_CM_2009]
• 低掺杂浓度下YAG:Ce有higher thermal quenching temperature。利用这一点可以制备YAG:Ce半透明(translucent)陶瓷，让掺杂浓度低一点，这样猝灭温度高，而厚的透明陶瓷可以让激发光通过更长的optical pathway，以弥补其相对于高掺杂浓度荧光粉吸收能力的差距。[Andries_CM_2009]

总结：[Thomas Jüstel-PPT]-P18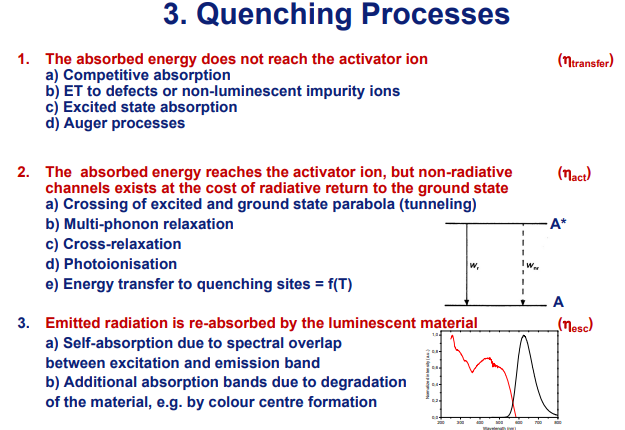

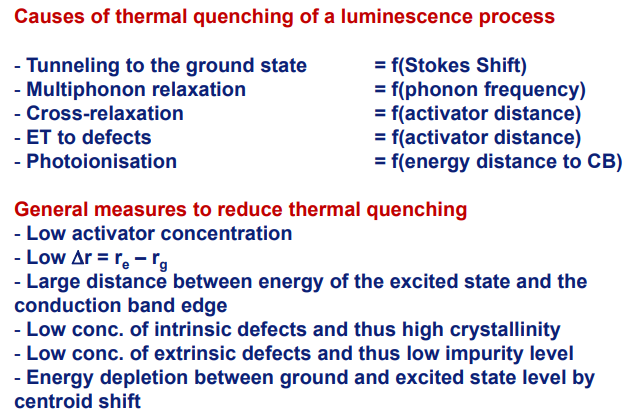
拓展阅读好文章：
(1) Unified Theory of Thermal Quenching in Inorganic Phosphors
(2) Thermal Quenching Analyses of Eu²⁺-Activated Sr-Containing Sialon Phosphors Using the Thermally Activated Cross-Over Model
(3) Insight into the Thermal Quenching Mechanism for Y₃Al₅O₁₂:Ce³⁺ through Thermoluminescence Excitation Spectroscopy
(4) On a blue emitting phosphor Na₃RbMg₇(PO₄)₆:Eu²⁺ showing ultra high thermal stability-JMCC-2019
(5) Non-quenching photoluminescence emission up to at least 865 K upon near-UV excitation in a single crystal of orange-red emitting SmPO₄-PCCP
(6) Predicting Thermal Quenching in Inorganic Phosphors-CM-2020

 

隧穿(Quantum tunnelling)效应

 

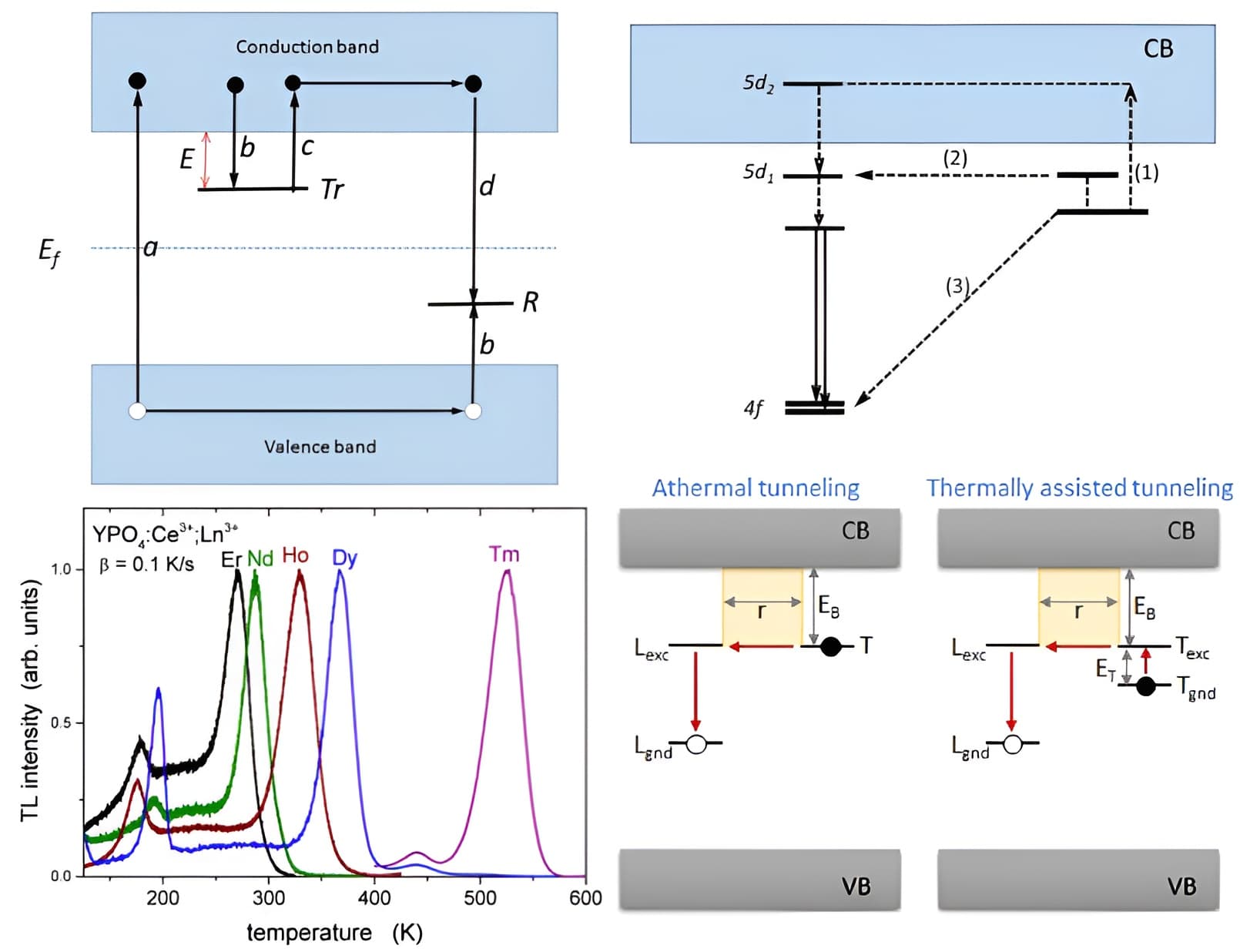左上图是simple model of TL, it is assumed that the recombination pathway of the electron goes via the CB. However, an alternative pathway is a localized transition where the electron recombines with a nearby hole center via s quantum tunnelling.
右上图Ce-doped Gd₃Al₂Ga₃O₁₂，(1) thermally stimulated non-localized transition (classical TSL), (2) thermally assisted tunnelling, and (3) ground state tunnelling recombination.
右下图来自[Tunneling recombinations in scintillators, phosphors, and dosimeters-Radiation Measurement-2018]，其他图和内容都来自[Thermoluminescence as a Research Tool to Investigate Luminescence Mechanisms- Adrie J. J. Bos-Materials-2017]

(1) Temperature Independent Quantum tunnelling
隧穿过程in principle是temperature independent，比如左下图的YPO₄:Ce³⁺,Ln³⁺, the Ce³⁺ ion is the stable hole center and the Ln-copants act as trapping centers. The plateau before the main TL glow peak looks like a background, but is in fact temperature independent luminescence caused by a localized transition. No “background” is observed on the high temperature side of the glow peak because the trap is then fully emptied.

Quantum tunnelling may be temperature independent but is critically dependent on the distance between the hole and trapping center. So one might expect that this plateau will rise as more hole and trapping centers are available, decreasing the average distance between the recombination center (Ce⁴⁺) and trapping center (Ln²⁺); in other words, as the concentration of the dopants increases.

(2) Thermally assisted Quantum tunnelling
Recombination pathway is via an excited state of the trapping center. 如右上图(2)过程，the population of the excited state is by thermal activation, causing the thermally assisted tunnelling to become temperature dependent。有人研究长余辉材料Ce-doped Gd₃Al₂Ga₃O₁₂，发现好几个不同温度的peaks都对应着差不多的trap depth，他们将其归因于electron  stored in oxygen vacancies recombine through a thermally assisted tunnelling mechanism with holes localized at Ce³⁺ centers residing on Lu sites at different crystallographic distances from the traps.

 

德拜温度(结构刚性)

计算：To investigate this point further, we calculated the Debye temperature (\( \Theta_{\mathrm{D}}\)) of RLSO with the DFT–PBE method, which was suggested as a useful proxy for structural rigidity. [夏志国_AM]

结构rigidity难以直接测试：一般来说，a large structural rigidity in the host materials should lead to a higher luminescence quantum yield. 和有机phosphor不同，无机晶体结构的rigidyity很难测量，因为晶体结构的各向异性以及复杂振动模式的存在。On the basis of Voigt-Reuss-Hill (VRH) approximations, the rigidity-related elastic parameters such as bulk modulus(体积弹性模量), shear modulus, Young’s modulus, and Poisson ratio can be easily obtained using elastic constants (\(C_{ij}\)) derived from DFT simulations. However, none of these elastic parameters can describe the degree of rigidity individually because of the versatile structural connectivity of inorganic lattices.

结构rigidity和德拜温度的关系：Given the positive correlation of the rigidity with the Debye temperature \( \Theta_{\mathrm{D}}\), which is the temperature required to activate the highest energy vibrations in a solid such as diamond and graphite, one can infer that the Debye temperature could serve as a useful probe for structural rigidity. Thus, it is reasonable to assume that the Debye temperature could be utilized for qualitatively estimating the quantum yield of the phosphors.

德拜温度和量子效率的联系：In principle, a high Debye temperature means inaccessible high energy phonon modes, resulting in a decreased number of nonradiative relaxation channels. Hence, it is reasonable to assume that such a temperature parameter can work for a broad spectrum of phosphors as the process of nonradiative relaxation is mainly responsible for luminescence quenching.

应用：As all elastic constants can be determined by direct computation, DFT calculations of Debye temperature could provide an effective way to screen vast databases of known inorganic structures in search for materials with a high probability of producing high quantum yield.

适用条件：This criterion appears to be valuable when no competing mechanism affects the performance of the 5d-4f luminescence, such as thermally activated escape of electrons from the d orbital to the host conduction band.

刚性和光谱振动结构：有理由认为，发光中心所处的刚性环境可以制约\(S\)和\(\Delta R\)值，使Stokes位移变小，有利于出现振动结构。(固体发光材料—P84)

文献Properties of Na₂SiF₆:Mn⁴⁺ and Na₂GeF₆:Mn⁴⁺ red phosphors synthesized by wet chemical用上了德拜温度拟合发光强度和温度之间的关系。

结构刚性和发光：(Suppression of Eu²⁺ Luminescence Loss-AOM-2022) Eu²⁺的发光受配位环境影响。Hence, it is intuitively projected that the loose structural framework of material will accommodate a strong lattice relaxation, which possibly results in the severe luminescence dissipation. This viewpoint makes sense for the analysis in the material incorporated by Ce³⁺ which owns an analogous 4f-5d luminescence as that of Eu²⁺, and it rationally interprets the remarkable luminescence performance of Ce³⁺ in compounds with dense structural frameworks. Also, stemming from this understanding, the strong struc-tural rigidity of lattice is conceived as the prerequisite for Ce³⁺ to acquire superior transition properties,  and this hypoth-esis has been accepted in the field and adopted to explain the advanced feature of phosphor frequently. 文中作者也举出高效Eu²⁺发光，但是结构不够刚性的例子。文中，作者还用红外/拉曼光谱、热容测试证实文章材料的没那么刚性。但是最后作者解释Eu2+发光强的原因是，weak electron-vibration interaction is the reason for the generation of narrow-band luminescence, which is essentially attributed to the highly rigid local coordination of Eu²⁺. This weak electron-vibration interaction also diminishes the nonradiative relaxation probability of excited Eu²⁺, and in combination with the experimental findings as collected from the X-ray spectroscopy, it proves that the deleterious impact of electron ionization on Eu²⁺ luminescence is suppressed, which consequently assures the superior luminescence efficiency of Eu²⁺-doped SMPO material.

基于rigidity”-controlled hypothesis，利用high-throughput computational screening，发现了新型 NaBaB₉O₁₅ :Eu²⁺荧光粉[Identifying an efficient, thermally robust inorganic phosphor host via machine learning-NC-2018]。

 

光电导/霍尔效应

• 通过光电导，可以看main charge carrier是电子还是空穴，从而证实是荧光粉是hole trapping还是electron trapping。
• 霍尔效应：磁场中运动的载流子受洛伦兹力作用，于是在极板上聚集形成电场(应该是材料放在溶液中？)，电场力与洛伦兹力达到平衡于是两极板间有稳定的电压。测试哪一侧为正极即可判断多数载流子是电子还是空穴。
• 光电导研究Ce³⁺的比较多，注意
  (1) 冯昂说透明陶瓷的，very shallow layer engaged in conductivity。
  (2) 制备透明陶瓷，粉末要细。

 

波长和能量转换&分峰

波长和电子伏特之间的关系为\(1024\)除以波长(nm)即为电子伏特的值。一般情况下从光谱仪测到的数据横轴的单位是nm，纵轴的单位是W/nm，注意纵轴的数值，是一个相对值，不同的不同的测试条件(固定波长)下的值是会有差异的，但是发射谱的谱形是相同的。积分面积的量纲显然就是W，即面积代表整个光谱的(相对)总功率。在将横轴从波长变为能量单位(比如波数，电子伏特等等)的时候，能量(或者说功率)是一个守恒量，那就必须保证\(f(\lambda)d\lambda=g(\nu)d\nu\)，其中\(f(\lambda)\)是原始数据，\(  g(\nu)\)是待求函数。根据转换关系\(\nu=\displaystyle\frac { 1024 }{\lambda } \)两边微分得到\( d\nu = -\displaystyle\frac { 1024 d\lambda }{\lambda^2} \)，将这个式子带入能量守恒式，得到\(f(\lambda)d\lambda=-g(\nu)\times \displaystyle\frac { 1024 d\lambda }{\lambda^2} \)，于是新得到的以频率单位为横轴的光谱函数$$g(\nu) =-f(\lambda)\times\lambda^2/1024$$

发射光谱的高斯分峰：比如ZnS:Cu呈现宽带发光峰，可以用两个高斯函数叠加而得到。特别注意分峰的时候横轴是频率\(\nu\)，纵轴是\(E(\nu)\)。

注意对于激发谱和吸收谱，从\(E(\lambda)\)到\(E(\nu)\)不需要Jacobian转换，直接用就行(只有发射谱需要转换)。[固体发光讲义-许少鸿]

David的文章中\(  \displaystyle\frac{\mathrm{d} \phi(E)}{\mathrm{d} E} \sim \lambda^{2} \displaystyle\frac{\mathrm{d} \phi(\lambda)}{\mathrm{d} \lambda}\)，实际上根据\(\displaystyle \int_{a}^{b} f(x) \mathrm{d} x=\displaystyle \int_{\alpha}^{\beta} f(x(u)) \displaystyle\frac{\mathrm{d} x}{\mathrm{~d} u} \mathrm{~d} u\)，有$$\int_{a}^{b} I(\lambda) \mathrm{d} \lambda=\displaystyle\int_{\alpha}^{\beta} I(\lambda(E)) \frac{\mathrm{d} \lambda}{\mathrm{~d} E} \mathrm{~d} E$$其中\( \lambda(E)=\displaystyle\frac { 1024 }{ E }\)，\(\displaystyle\frac{\mathrm{d} \lambda}{\mathrm{d} E}=-\displaystyle\frac { 1024 }{E^2 }\)，代入之后得。

Researchgate: Which is the right approach to convert from wavelength to energy scale taking into account the monochromator dispersion effect for optical measurements?

 

 

Periodic table of the "lighting" elements

来自[New developments in the field of luminescent materials for lighting and displays.-Angew-1998] 后面又陆续加入了Te、Ho。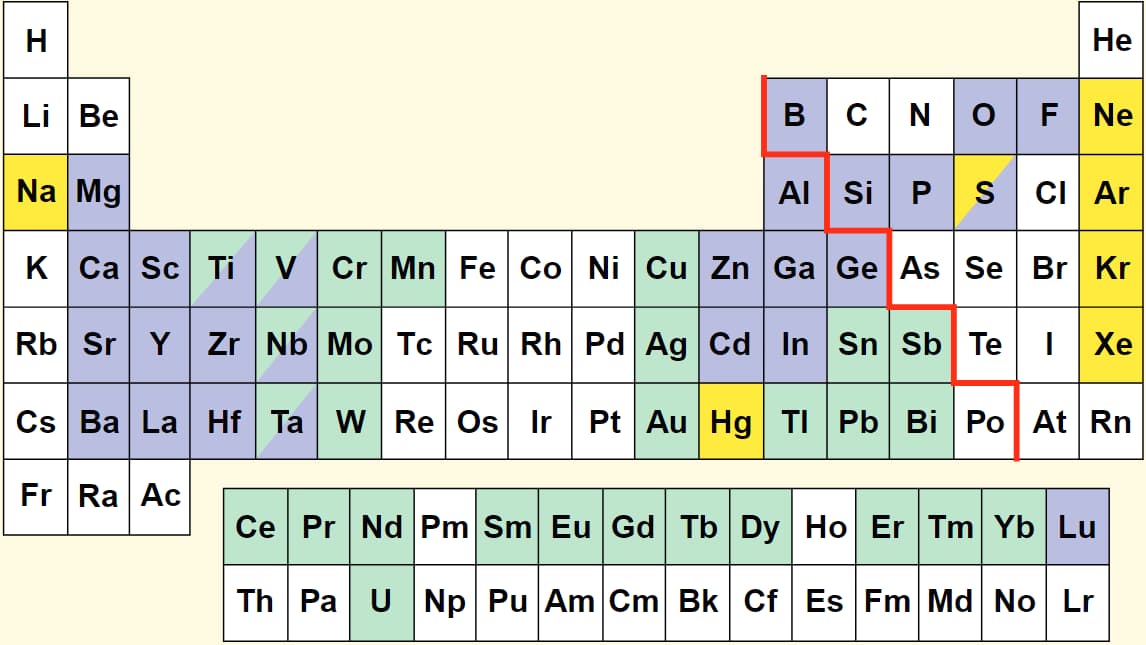

这些激活离子和等离子元素，都可作为冷光源中跃迁中心，实现发光。所谓冷光源(luminescence)主要是区别于热辐射光源。冷光源的发光原理基本都是基于电子跃迁，所以比如说荧光，LED还有激光都属于冷光源。类似冷光源的概念，还有“冷热源”，微波加热是一种“冷热源”，它在产生和接触到物体时，不是一股热气，而是电磁能’ 它具有一系列传统加热所不具备的独特优点(即时性、整体性、选择性、高效性、杀菌等)

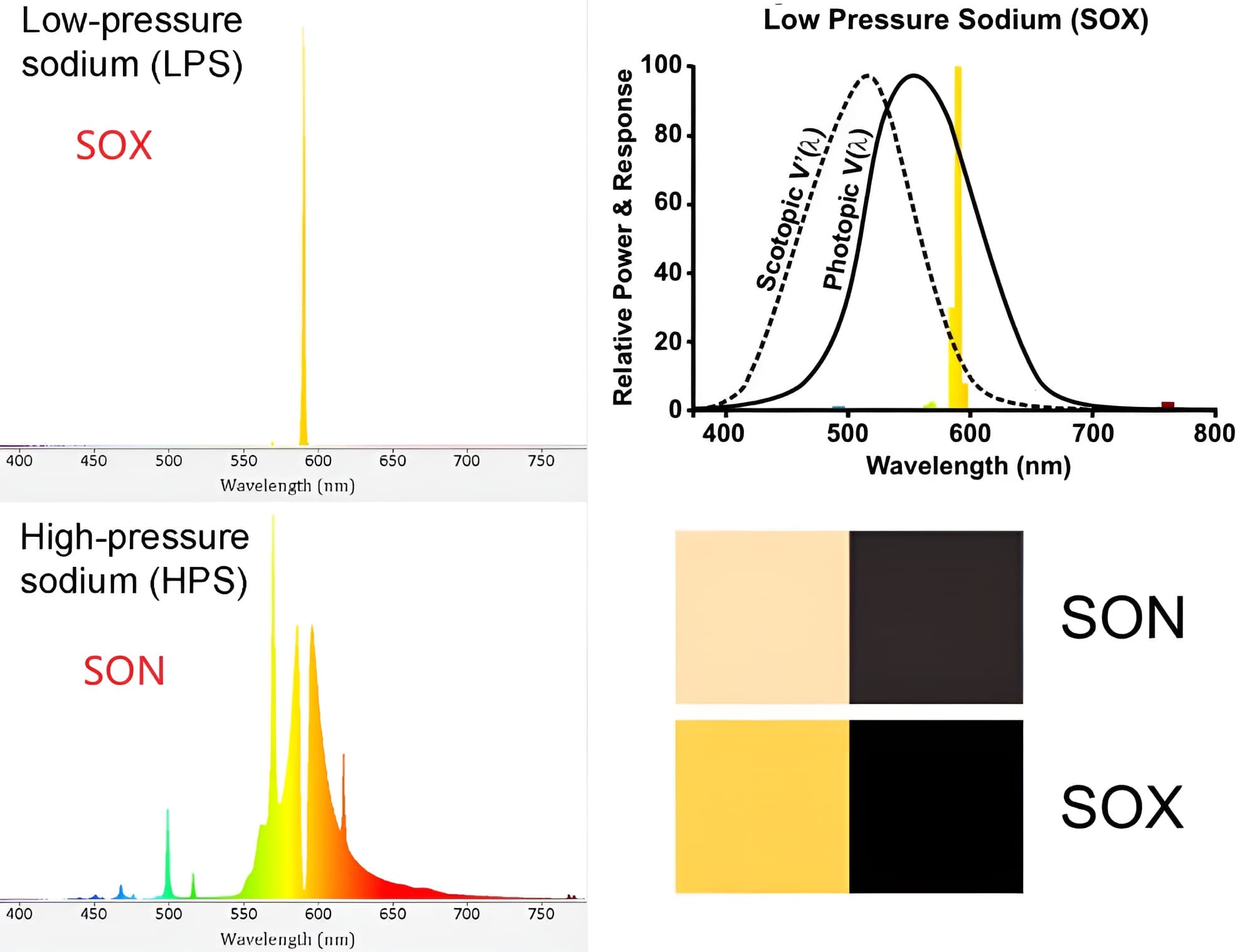
钠灯：钠灯的灯管内也会充填汞和稀有气体，但实际上起作用的是钠蒸气。钠被电离、激发后会发射出589nm的黄色光线，这些光线直接用于照明。
(1) 低压钠灯：工作时其电弧管内的蒸汽压为0.7～1.5 Pa，光近乎单色，集中在589 nm和589.6 nm，对人眼较敏感的黄光区域，所以发光效率高达150流明/瓦以上，但显色性太差，只用于不需分辨颜色的场合。可作为旋光仪、折射仪、偏振仪等光学仪器中的单色光源；
(2) 高压钠灯：提高钠的蒸汽压，并加入少量汞，光线的谱线更宽，所以显色性比低压钠灯好，色温为2100开，光效为72～130帕流/瓦，是道路照明的主要光源，也用于舞台等场合的照明。发光颜色为金白色光，它具有发光效率高、耗电少、寿命长、透雾能力强和不诱虫等优点。广泛应用于道路、高速公路、机场、码头、船坞、车站、广场、街道交汇处、工矿企业、公园、庭院照明及植物栽培；
(3) Blacks appear blacker under SOX light - contrast is enhanced.

参考资料：
(1) 为什么汽车雾灯用黄光 而不是醒目的红光？
(2) Spectral Properties of the Sodium Discharge

注：
霓虹灯-Wiki